Recent presentations:
Poster, for HRS 2009 meeting in Boston

Human ventricular cell (TNNP) model

Recently we introduce a mathematical model of the action potential of human ventricular cells that, while including a high level of electrophysiological detail, is computationally cost-effective enough to be applied in large-scale spatial simulations for the study of reentrant arrhythmias (see figure below). The model is based on recent experimental data on most of the major ionic currents and reproduces properties of different cell types (endocardial, epicardial and M cells), the experimentally observed data on action potential duration restitution and the conduction velocity restitution. We use this model to study dynamics of spiral wave rotation in 2D and in anatomically accurate model of human heart.

( K.H.W.J. ten Tusscher, D. Noble, P.J. Noble, and A.V. Panfilov, 2004, American Journal of Physiology, 286, H1573-H1589. (Full text (HTML) and PDF for AJP subscribers); PubMed ID: 14656705)

( New version: K.H.W.J. ten Tusscher and A.V. Panfilov, 2006, American Journal of Physiology, 291(3):H1088-100. (Full text (HTML) and PDF for AJP subscribers); PubMed ID: 16565318)

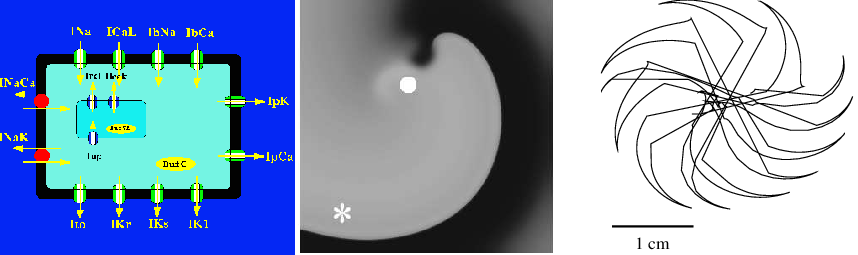
 Schematic representation(left);     Spiral wave dynamics (middle)                Core of spiral wave (left)

Links

The webpage of K. Ten Tusscher, including PhD thesis

The source codes

Cell ML implementation

Anatomically accurate modelling

   

---

canine ventricular model

---

Another direction of studies is the development of anatomically based models of heart ventricles. In 1992-1994 we developed an anatomically based model of ventricles of canine heart ( Panfilov and Keener, Chaos Solitons and Fractals,v.5,p.681-689,(1995), A.V.Panfilov, in ''Computational Biology of the Heart'. ed. A.V. Panfilov and A.V. Holden, Wiley,p.259-276, (1997)). The model was based on anatomical data of geometry and fiber orientation obtained by the group of P. Hunter from Auckland University. The dynamics of cardiac cells was represented by means of a FitzHugh-Nagumo model, where a diffusion matrix accounts for tissue anisotropy and fiber orientation. Using this model normal and abnormal excitation on the heart was studied: three-dimensional re-entrant behavior resulting from single or multiple re-entrant sources, re-entry initiation due to a single premature stimulus, and ventricular fibrillation due to the process of spiral breakup. The patterns of excitation perdicted by this model were later recoded in experiment during ventricular fibrillation in dog heart ( Witkowski et al., Nature,v.392,p.78,1998). Later, that patterns were quantified and three dimensional sources of excitation driving ventricular fibrillation were counted ( A.V.Panfilov, Phys. Rev.E, v.59, p.R6251-R6254, (1999).

---

Movies of wave propagation in a model of dog heart

just click on picture

HUMAN VENTRICULAR MODEL together with Olivier Bernus and Kirsten Ten Tusscher

---

Starting form 1997 we are developing an anatomically accurate model for ventricles of human heart based on anatomical data set by R. Hren and our equations for human cardiac cells. We use this model to study menifestation of different arrhythmias and ventricular fibrillation in human heart,

---

Movies using gamma model for human ventricular cells

---

---

Movies using TNNP model for human ventricular cells

---

---

Movies of wave propagation in a model of human atria (work in progress with Ch.Zemlin )

---

---

Reactio-diffusion-contraction systems (work in progress with M.Nash )

---

---

left -Self-Organized Pacemakers due ot mechanical activity
middle-left -mechanical activity during spiral breakup
middle-right -Breakup induced by mechanical activity
right -Spiral in anatomical model of pig heart (in progress)

---

Nash MP, Panfilov AV. : Prog Biophys Mol Biol. 2004 Jun-Jul;85(2-3):501-22.

Panfilov AV, Keldermann RH, Nash MP.:Phys Rev Lett. 2005 Dec 16;95(25):258104

Panfilov AV, Keldermann RH, Nash MP.:Proc. Natl. Acad. Sci. USA, 2007, v.104,p.7922-7926